If you think of length as being one dimensional, that is, going in one direction only, then area is two dimensional, because it has length and width.

Let's have a look at the area of some common shapes.

Squares and rectangles

The area of any square or rectangle is simply its length times its width. For example, if a rectangle is 3 metres long and 2 metres wide, its area is:

      Length x width = 3 m x 2 m = 6 square metres (m²)

What if you had a sheet of particleboard measuring 3.6 m x 1.8 m? Its area is simply:

      Length x width = 3.6 m x 1.8 m = 6.48 m²

Triangles

Let's say you cut the sheet of particleboard in half diagonally, forming two equal triangles. The area of each triangle is exactly half of the original rectangle. That is:

      Length x height ÷ 2 = 3.6 x 1.8 ÷ 2 = 3.24 m²

This proves that a triangle is half the area of the rectangle or square that it came from.

So even if you had a triangle that didn't have a right angle in it, the calculation is still the same, because you could simply divide the triangle into 2 triangles, and the rectangle around it into 2 rectangles.

But note that you must always measure the height of the triangle at right angles (90 degrees) to the base.

You can't measure the diagonal line in the triangle, because that's not the true height of the rectangle that goes around it.

Circles

You may remember from your school days that the formula for the area of a circle is: Π r², where Π is 3.14, and 'r' is the radius of the circle.

If you're happy using that formula you can stay with it, but you might prefer this simplified version - which is actually the same, but just put in different terms:

     Area of a circle = diameter x diameter x 3.14
                                  2             2

Another way of writing this is:

     Area = (diameter ÷ 2) x (diameter ÷ 2) x 3.14

Here's an example. If a circle is 1.2 m in diameter, what's its area? The answer is shown below.

Area = (diameter ÷ 2) x (diameter ÷ 2) x 3.14

       =   (1.2 ÷ 2)       x       (1.2 ÷ 2)       x       3.14

       =   0.6       x       0.6       x       3.14

       =   1.13 m²

So where does 3.14 come from? This is actually the approximate ratio between the circumference, or outside measurement, of the circle and its diameter.

In other words, the circumference of a circle is roughly 3.14 times longer than its diameter.

Compound shapes

If you can break a shape up into its basic parts, you can calculate its area by adding the separate areas together.

Example 1: L shape

This L shape is basically two rectangles. What is its area? Note that the measurements in the diagram are shown in millimetres, so you'll need to convert them into metres for the calculation. A good way to set out the workings is as follows.

          Rectangle 1:   1.9 x 0.85 = 1.615 m²

          Rectangle 2:   0.95 x 0.85 = 0.808 m²

          Total area:   1.615 + 0.808 = 2.423 m²

Written mathematically, this would be:

          (1.9 x 0.85) + (0.95 x 0.85) = 2.423 m²

Example 2: Gable end of a house

This shape is a triangle plus a rectangle. Again, you can set out your workings in the same way.

          Triangle: 1.59 x 4.125 ÷ 2 = 3.279 m²

          Rectangle: 2.75 x 4.125 = 17.05 m²

          Total area: 5.58 + 17.05 = 22.63 m²

Written mathematically: (1.8 x 6.2 ÷ 2) + (6.2 x 2.75) = 22.63 m²

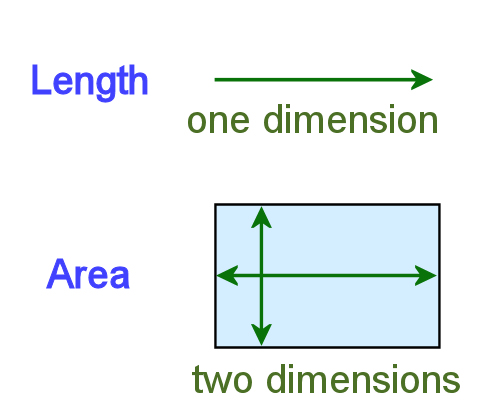
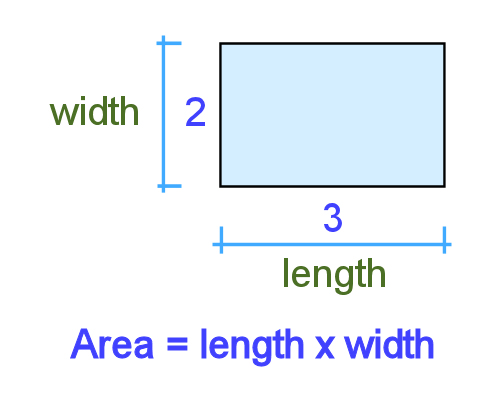
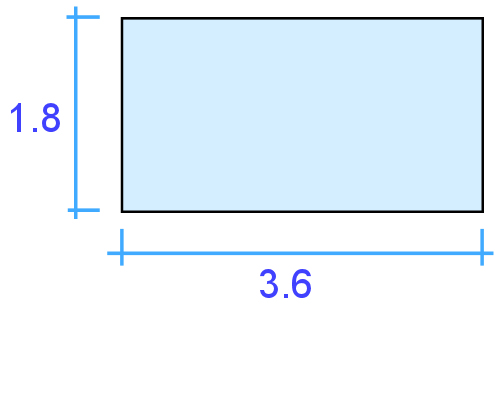
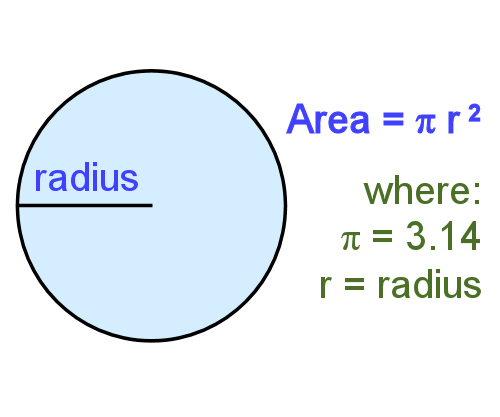
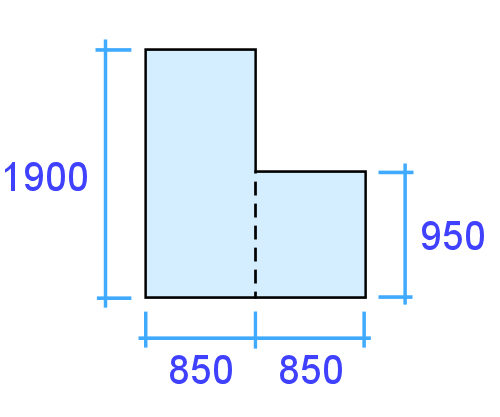
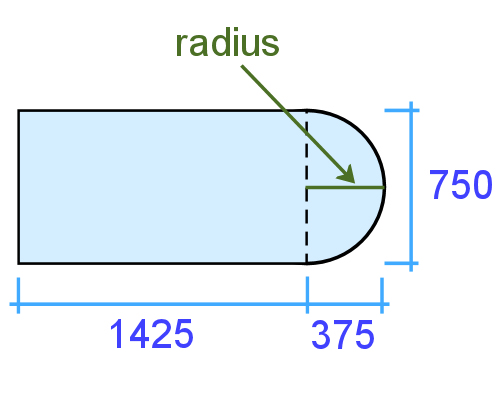
Example 3: Kitchen bench top

This shape is half a circle plus a rectangle. We know that the diameter of the circle is 750 mm, because that's the width of the bench.

Therefore we know that the curve begins at 1425 mm along the bench, because the radius of the circle is half of the diameter. That is:

        Radius = 750 ÷ 2 = 375 mm.

The area of the semicircle is simply half the area of the whole circle. So now you can work out the two separate areas and add them together, as shown below.

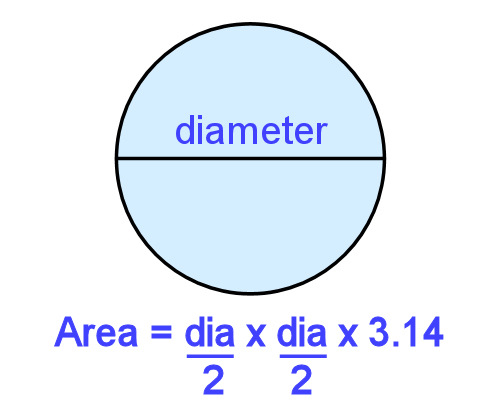
Semicircle     = (diameter ÷ 2) x (diameter ÷ 2) x 3.14 ÷ 2

                  = (0.75 ÷ 2) x (0.75 ÷ 2) x 3.14 ÷ 2

                  = 0.375 x 0.375 x 3.14 ÷ 2

                  = 0.22 m²

Rectangle: 1425 x 750 = 1.069 m²

Total area: 0.22 + 1.069 = 1.289 m²

Written mathematically: (0.75 ÷ 2 x 0.75 ÷ 2 x 3.14 ÷ 2) + (1.425 x 0.750) = 1.289 m²